Let [f(x) = xn]. By definition, the derivative would be:
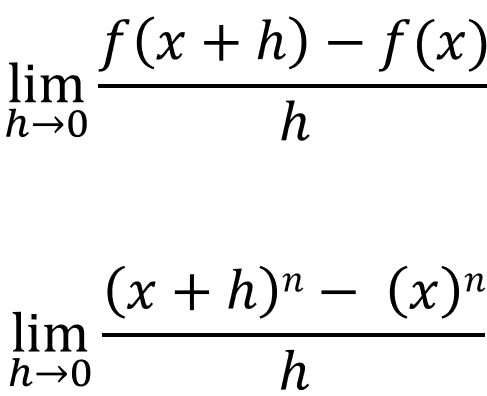
If we expand (x+h)n using the binomial theorem, we get:
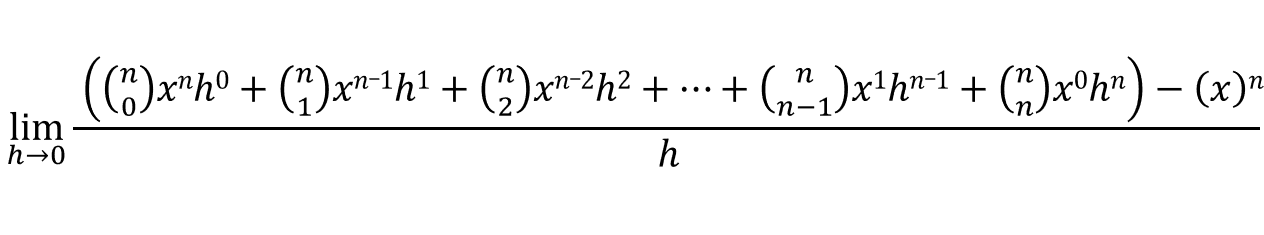
In the numerator, the first and the last terms would cancel out:
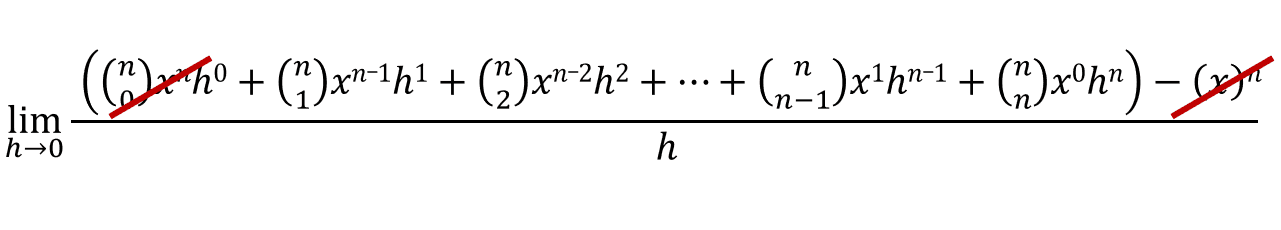
Now if you look at the numerator, the variable h exists in every term one or more times, this means we can factor out one h from every term in the numerator:
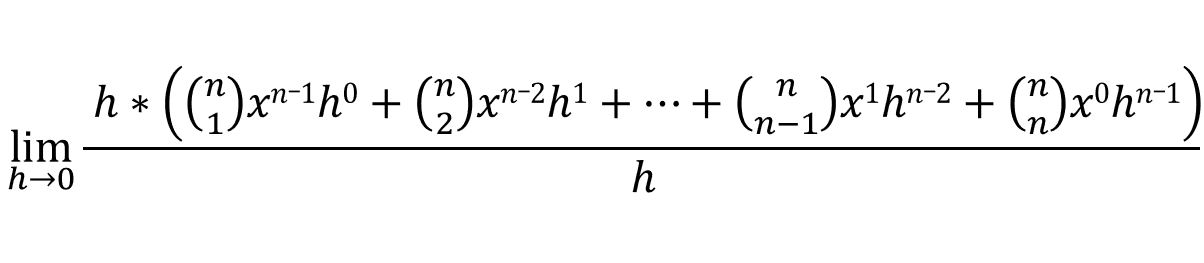
If we simplify, we get:
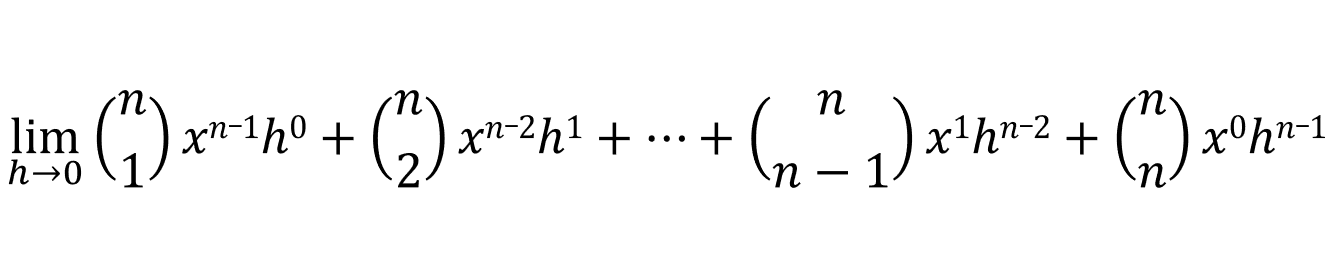
Any number to the power of zero is one as long as that number is not zero, since h goes close to zero but doesn't equal it, then [h0 = 1]:
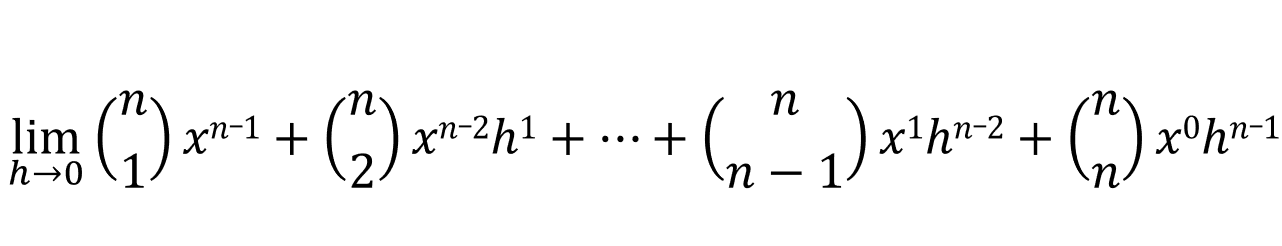
There is one rule of limits is which states that [limn->0 n = 0]. If every term (except for the first one) has the h variable and if h goes closer to zero, then that means all the terms except for the first one will approach zero:
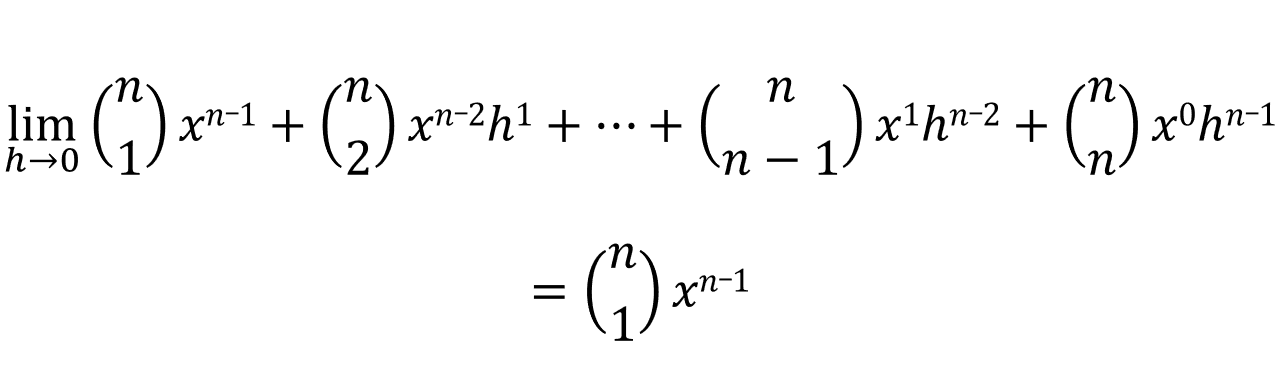
Since nC1 will always be equal to n (as long as n is an integer greater than one) this means that:
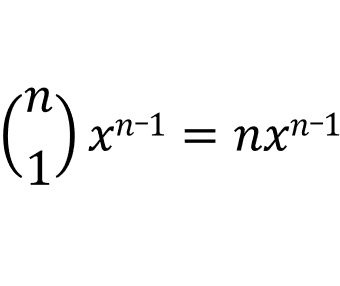
Hence, we have proven that [d/dx](x^n) = nx^(n-1) when n is an integer greater than one.